ในรายงานนี้ จะเสนอผลการจำลองแบบภาพปรากฏที่เกิดขึ้น โดยรังสีจากแหล่งกำเนิดที่เป็นจุด สะท้อนผ่านกระจกเว้า แล้วหักเหจากเกรติงระนาบแบบสะท้อน ภาพปรากฏนี้ได้จากการคำนวณเส้นทางของรังสีโดยกฎการสะท้อน และสมการการเลี้ยวแบนจากเกรติงแบบฉบับ แต่เนื่องจากภาพที่เกิดขึ้นอยู่นอกแนวแกนมุขสำคัญของกระจกโค้ง และแนวลำแสงที่ตกลงบนเกรติงไม่ขนานกัน รูปแบบของภาพปรากฏจึงไม่อาจคาดเดาได้โดยง่าย จากการจำลองแบบพบว่า ไม่สามารถโฟกัสภาพปรากฏลงบนจุด แต่สามารถบีบแนวลำแสงให้อยู่บริเวณขอบของภาพได้ โดยการวิเคราะห์การแจกแจงความเข้มบนภาพปรากฏ สามารถกำหนดตำแหน่งที่เหมาะสม ที่อาจใช้เป็นตัวแทนของเส้นสเปกตรัมของแหล่งกำเนิด ข้อมูลที่ได้จะเป็นประโยชน์ในการออกแบบสเปกโตรมิเตอร์แบบเกรติงระนาบ ซึ่งเป็นอุปกรณ์ที่หน่วยปฏิบัติการวิจัยฯ กำลังดำเนินการพัฒนาขึ้นอยู่ในขณะนี้

Introduction: In recent years, from the needs of optical technology development, light source characterization is of considerable importance in optical metrology. The basic instrument for the characterization process is the grating spectrometer, which can separate polychromatic light into its constituent monochromatic components. In our research unit, we are currently developing the Monk-Gillieson type, plane grating spectrometer. This development is a part of our CCD spectrograph project, which we try to develop grating spectrometer that use the CCD to sense the spectrum images from the light sources. The reason we choose the Monk-Gillieson type because it uses least optical elements as possible. The basic principle of this spectrometer is not complicated, but building this kind of instrument that can reach the standard is not simple. One of the most important problems is the optimization of device configuration that maximizes the resolution. However, this topic is rarely met in ordinary text book in optics. Since the images are not formed on the principle axis of the mirror and the incident rays on grating are not parallel, the forms of images are not obvious. In this paper, we have simulated the images from the spectrometer, using the simple model of concave mirror and plane reflecting grating. From the resulting images, intensity distribution can be analyzed. These data are expected to be useful in locating the appropriate positions that represent the spectrum of the source, which are the most important data needed in the designing of the spectrometers.

Methodology: Rays emerged from a point source were reflected at a concave mirror. The directions of the reflected rays were calculated using simple rule of reflection. These rays were then reflected again from a plane reflection grating. The directions of the diffracted rays were calculated from the conical grating equation:

Here G is the groove density, m is the diffraction order, ? is the angle between the incident light path and the plane perpendicular to the grooves at the grating center, and ? and ? are the angles between the grating normal and the projections of incident and diffracted rays on the plane perpendicular to the grooves. As shown in figure 1, grating was placed at the same plane with the source to minimize the tilt of the mirror. The images were measured on the image planes where the sensing devices were assumed to be placed. From the resulting images, intensity distributions on the axis of symmetry were analyzed by dividing the axis to intervals and counting the number of rays fell on them.

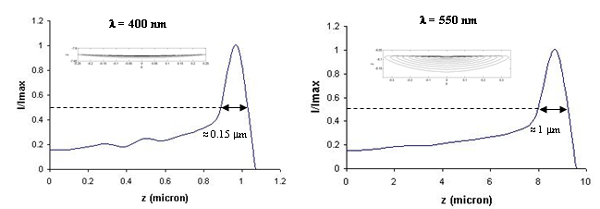
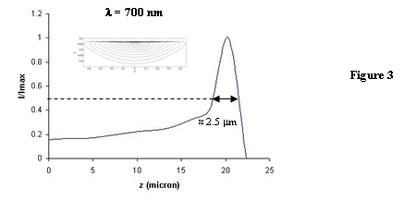
Results, Discussion and Conclusion: Due to our preliminary design, the following parameters were used: radius of curvature of concave mirror R = 18 cm, diameter of the mirror d = 4.83 cm, distance from point source to mirror L = 13.50 cm, tilt angle of the mirror = 6? (the minimum angle that the reflected ray did not overlap with the edge of the pinhole, which was used as the point source), and groove density G = 600 lines/mm. Figure 2 shows the images of the 550 nm point source at various positions. Off axis aberrations were observed. It was found that the images could not be focused on a point but could be squeezed at the rim. Figure 3 shows such the images of the point sources with various wavelengths, together with the corresponding intensity distributions on the axis of symmetry. The width of the intensity signals are approximately 1-5 m, which match the pixel size of the CCD sensor used in our project. These squeezed lines can be used to represent the spectrum of the source. The simulation results are the useful data in the designing of the plane grating spectrometers which are currently developed in our research unit. However, we hope that these data may be useful in general for student and researcher who are interested in the topics of optics. For the interested reader, source code that used to produce these results can be downloaded from our web site at http://labnet.sci.ku.ac.th/ .